This page was generated from `/home/lectures/exp3/source/notebooks/L1/Geometrical Optics.ipynb`_.
Binder badge

Geometrical Optics

Geometrical optics refers to a field of optics that describes light propagation in terms of rays. This is on one side borrowed from your daily experience, for example, with shadows. On a more scientific side, it will turn out that geometrical optics is the approximation in which the wavelength of light is much shorter.

Geometrical Optics

Geometrical optics is an approximate description of light propagation in the limit of infinitely small wavelength, where all wave phenomena like diffraction can be neglected.

Assumptions

As geometrical optics is not a rigorous description, we have to write down some postulates (things we do not understand yet) to describe light propagation in geometrical optics

  • light rays emerge from a light source

  • light rays are detected by a detector

  • light propagates in straight line paths (rays) in a homogeneous medium

  • light–matter interaction is characterized by a refractive index \(n\)

  • light bends to a curved path in inhomogeneous media (i.e., \(n(\vec{r})\))

  • rays may be reflected and refracted at interfaces between media

Let us have a look at some examples providing indications for the linear propagation of light.

Laser

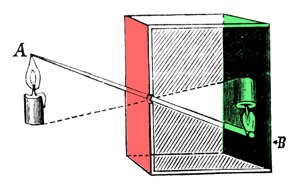
Pinhole Camera

9340e2635a774278860cfec37b3e2c4a

Fig.: Schematics of a pinhole camera.

4ddb593f98c14bfd8859790181684da6

Fig.: Image of an upright letter “F” on the screen of a pinhole camera in lecture 1.

8015c8ff3b4e47b3b334960e1bfbfb33

Fig.: Core shadow and partial shadows behind a disk when illuminated with two seperate light sources.

Water Basin with Salt

6be40ee4f9da4106aa8a6d6c3e5e6cd6

Fig.: Bend rays in a refractive index gradient with a salt (NaCl) layer on the bottom.